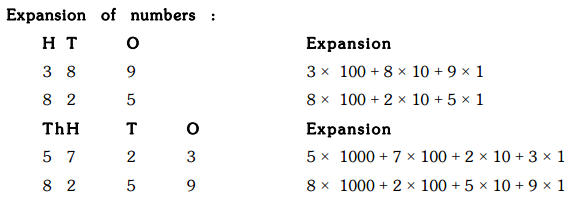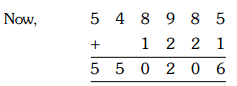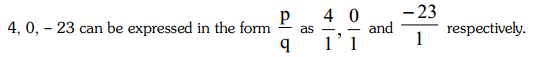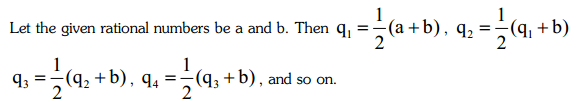CBSE Archives - WorkSheets Buddy

An Angel in Disguise Summary in English by T.S Arthur

December 1, 2022November 30, 2022 by Prasanna

An Angel in Disguise Story Summary in English and Hindi Pdf. An Angel in Disguise is written by T.S Arthur.

An Angel in Disguise Story Summary in English by T.S Arthur

An Angel in Disguise About the Author

T.S. Arthur who was born on June 6, 1809 had little formal education. He used to hear stories from the Bible and stories about his grandfather who was an officer in the Revolutionary War, from his mother. He was fond of reading books and thus educated, himself through reading. Later he became an apprentice for a Baltimore craftsman.

Later on he became an editor for the Baltimore Athenaeum and Young Men’s Paper. Three years later he started the Baltimore Literary Monument. It was during this period that Arthur learned of the Washingtonian Temperance Society, which inspired him to write many novels on prohibition.

His novels were such a success that during the decade after the Civil War, the only author that outsold him in American fiction was Harriet Beecher Stowe with Uncle Tom’s Cabin.
The older he grew the worse his eyesight became. His world narrowed during the early years of the 1880s, although he continued editorial work with the aid of amanuenses. By February 1885, he was unable to leave his home and died on March 6th, 1885. He was buried in Philadelphia’s old Chestnut Street Cemetery.

An Angel in Disguise About the Story

All about the Story :
The author stresses the importance of loving and caring in the story ‘An Angel in Disguise’. He believes that it is essential for human survival. He explains the true meaning of compassion, kindness and love very clearly.

In this story, T.S. Arthur strikes a didactic note by laying emphasis on the importance of loving and caring for others. He points out that love is essential for human survival and happiness.

The story is about a poor woman who falls upon the threshold of her own door in a drunken fit and dies leaving behind two daughters and a son to fend for themselves. The townspeople take pity on these children and the two oldest are taken in by new families, but the youngest Maggie, who is crippled, is left alone because no one wants to deal with her disability. A man named Joe Thompson decides to take her in for the night but plans on taking her to the poorhouse the next morning, because he knows his wife would not approve of her. When Joe brings Maggie home in arms, his wife is enraged that he brings that ‘sick brat’ into her house.

Joe begs her : “Look at her kindly, Jane; speak to her kindly.” “Think of her dead mother, and the loneliness, the pain, the sorrow that must be on all her coming life.” The softness of his heart gives eloquence to his lips. While Joe is out at work, his wife spends the day with Maggie and grows very fond of her.

The Thompsons end up keeping Maggie and she becomes a blessing. “It had been dark and cold and miserable there for a long time just because his wife had nothing to love and care for out of herself, and so became sore, irritable, ill-tempered and self-afflicting in the desolation of her woman’s nature. Now the sweetness of that sick child, looking ever to her in love, patience and gratitude, was as honey to her soul, and she carried her in her heart as well as in her arms, a precious burden.” Maggie was an angel in disguise.

An Angel in Disguise Summary in English

Death of a poor woman
The story ‘An Angel in Disguise’ tells us about a poor woman who was in the habit of drinking. Once she was in a drunken fit and therefore fell upon the threshold of her own door. She died leaving two daughters and a son behind to fend for themselves. This woman had been despised, scoffed at, and angrily denounced by nearly every man, woman and child in the village. But now people took pity on her. Neighbours went hastily to her hut with food for the half-starving children, three in number.

Of these, John, a boy of twelve, was a stout lad. Kate, between ten and eleven, was a bright, active girl; but poor little Maggie, the youngest, was hopelessly diseased. Two years before, a fall from a window had injured her spine and so she was bed-ridden. The townspeople pitied these children, and the two oldest were taken in by new families, but the youngest Maggie, who was crippled, was left alone because nobody wanted to deal with disability. One of them said, “Take her to the poorhouse”. But another said, “The poorhouse is a sad place for a sick and helpless child.”

Joe Thompson takes pity on Maggie
A man named Joe Thompson, the wheelwright, took pity on Maggie and decided to take her with him for the night but planned to take her to the poorhouse the next morning, because he knew his wife would not approve of her. Joe was a kind man and liked children. He lifted her in his strong arms and took her to his home. Now his wife, who was childless, was not a woman of saintly temper. She saw her husband approaching from the window. When Joe entered his home with Maggie in his arms, his wife was enraged and said, “You have brought home that sick brat !” Anger and astonishment were in the tones of Mrs Joe Thompson and her face was in flame. Joe did not reply except by a look that was pleading and cautionary, that said, “Wait a moment for explanation and be gentle.” Joe explained to her that after her mother’s funeral, everybody went away. She was left alone in her hut. So he brought her here. He told her that he would take her to the poorhouse the next day. She told him to go at once and leave her there.

Children need Special care
“Jane”, said Joe, with an impressiveness of tone that greatly subdued his wife, “I read in the Bible sometimes, and find much said about little children. How the Saviour, rebuked the disciples who would not receive them, how he took them up in arms and blessed them, and how he said that ‘whosoever gave them a cup of cold water should not go unrewarded’. Now, it is a small thing for us to keep this motherless little one for a single night, to be kind to her for a single night to make her life comfortable for a single night.” The voice of the strong, rough man shook, and he turned his head away, so that the moisture in his eyes might not be seen. Mrs Thompson did not answer, but a soft feeling crept into her heart.

“Look at her kindly, Jane, speak to her kindly,” said Joe. “Think of her dead mother, and the loneliness, the pain, the sorrow that must be on all her coming life.” The softness of his heart gave eloquence to his lips.

A change in Joe’s wife
While Joe was out at work, his wife spent the day with Maggie. When Joe returned he saw Maggie lying a little raised on the pillow with the lamp shining full upon her face. Mrs Thompson was sitting by the bed, talking to the child. He was happy to see a change in his wife’s attitude. A deep-drawn breath was followed by one of relief, as a weight lifted itself from his heart. Joe entered the house but did not go immediately to the little chamber. His heavy tread about the kitchen brought his wife suddenly from the room where she had been with Maggie. Joe thought it best not to refer to the child.

“How soon will supper be ready ?” he said. “Right soon”, answered Mrs Thompson with no roughness in her voice. After washing his hands and face, he went into the little bedroom where Maggie was lying. He looked at Maggie and saw that it had a lovely face.

“Your name is Maggie ?” he said, as he sat down and took her soft little hand in his..

“Yes, sir.” Her voice struck a chord that quivered in a low strain of music.

“Have you any pain ?” said Joe.

“Sometimes, but not now.” replied Maggie. Mrs Thompson takes care of Maggie

They continued conversing with each other. Then Mrs Thompson came and said that supper was ready. Joe glanced from his wife’s face to Maggie’s. She understood him, and said, “She can wait until we are done, then I will bring her something to eat.” After sometime, she said, “What are you going to do with that child ?” “I thought you understood me that she was to go to the poorhouse,” replied Joe. Mrs Thompson gave food to Maggie and asked her if it was good. The child answered with a look of gratitude that awoke to new life old feelings which had been slumbering in her heart for half a score of years.

“We’ll keep her a day or two longer, she is so weak and helpless,” said Mrs Joe Thompson.

Maggie – a blessing : Mrs Thompson grew very fond of Maggie. The Thompsons ended up keeping Maggie, and she became a blessing. “It had been dark, and cold and miserable there for a long time just because his wife had nothing to love and care for out of herself, and so became sore, irritable, ill-tempered, and self-afflicting in the desolation of her woman’s nature. Now the sweetness of that sick child, looking ever to her in love, patience and gratitude, was as honey to her soul, and she carried her in,her heart as well as in her arms, a precious burden.” Maggie was an angel in disguise.

An Angel in Disguise Word Notes and Explanations

intemperance – drunkenness/ self-indulgence
wretched – bad
despised – disliked
scoffed at – mocked at
denunciation – criticism
charitable – kind
attire – dress
puzzled – confused
wheelwright – A person whose job is making and repairing wheels
precious – valuable
astonishment – surprise
countenance – a person’s face
indignation – a feeling of anger
tossed – threw
rebuked – scolded
tread – walk
asperity – roughness
obliterate – remove
mingled – mixed
slumbering – sleeping
almshouse – a house owned by charity
miserable – unhappy
irritable – getting annoyed
desolation – loneliness
gratitude – feeling of being grateful
disguise – concealment
dreary – dull

An Angel in Disguise Theme

In this story ‘An Angel in Disguise’, the author strikes a didactic note by laying emphasis on the importance of loving and caring for others. He argues that love is essential for human survival and happiness. Through this story, he points out the true meaning of compassion, kindness and love. He conveys his idea through the story of a poor woman who falls upon the threshold of her own door in a drunken fit and dies leaving behind two daughters and a son to fend for themselves. The townspeople take pity on these children and the two oldest are taken in by two families, but the youngest, Maggie, who is crippled, is left alone because no one wants to deal with her disability.

A man named Joe Thompson decides to .take her in for the night but plans on taking her to the poorhouse the next morning, because he knows his wife would not approve of her. When Joe hrings Maggie home in arms, his wife is enraged that he brings that ‘sick brat’ into her house.
Joe begs his wife to treat her kindly. He tells her to think of her dead mother, and the loneliness, the pain and the sorrow that must be on all her coming life. He refers to the Bible in which the Saviour has taught his disciples to love children.

While Joe is out at work, Mrs Thompson spends the day with Maggie and develops compassion for her. Then she grows fond of her. The Thompsons end up keeping Maggie and she becomes a blessing. It had been dark and cold and miserable there for a long time just because she (Thompson’s wife) had nothing to love and care for except herself and so became sore, irritable, ill-tempered and self-afflicting in the desolation of her woman’s nature. Now the sweetness of that sick child, looking ever to her in love, patience and gratitude, was like honey to her soul, and she carried her in her heart as well as in her arms, a precious burden. Maggie becomes an angel in disguise.

The writer wants to convey that not only does Maggie need the care of another to survive, but Mrs Thompson also needs Maggie as someone to care and love for, to live a happy purposeful life. Mrs Thompson gets a direction to lead a pleasant life.

An Angel in Disguise Title

The title of the story ‘An Angel in Disguise’ is most appropriate because the story deals with Maggie, the youngest of her family, who proves to be an angel in disguise for the Thompsons. A poor woman falls upon the threshold of her own door in a drunken fit and dies leaving behind two daughters and a son to fend for themselves. The two oldest children – John and Katy – are taken away by new families but the youngest Maggie, who is crippled, is left alone because no one wants to deal with her disability. Joe Thompson, a kind-hearted person, takes Maggie to his home. His wife Mrs Thompson is enraged to find that he has brought that ‘sick brat’ to her home.

Joe Thompson exhorts his wife to be kind to her and treat her gently. But Mrs Thompson, who happens to be childless, is an irritable and ill-tempered woman. She spends a day with Maggie. Gradually, she grows fond of her and begins to love Maggie. The sweetness of Maggie, the sick and helpless child, looking ever to her in love, patience and gratitude, is like honey to her soul, and she carries her in her heart as well as in her arms, a precious burden. Maggie’s love has completely conquered Mrs Thompson and she is totally changed into a kind, caring and loving woman. Maggie, for Mrs Thompson, is an angel in disguise. Thus the title is apt and suitable.

An Angel in Disguise Message

In this story ‘An Angel in Disguise’ the writer gives a moral lesson. He lays emphasis on the importance of loving and caring for others. He proves that love is essential for human survival and happiness. Through this story, he points out the true meaning of compassion, kindness and love. He conveys this idea by depicting the story of a poor woman who falls upon the threshold of her own door in a drunken fit and dies leaving behind two daughters and a son to fend for themselves. The townspeople take pity on the children and the two eldest are taken away by two families, but the youngest, Maggie, who is crippled, is left alone because no one wants to deal with disability.

A man named Joe Thompson takes pity on her and decides to take her to his house for the night. He plans to leave her in the poorhouse the next morning because he knows that his wife would not approve of her. The same thing happenned. When Joe brings her home in his arms, his wife is enraged that he has brought that ‘sick brat’ into her house. Joe, the mouthpiece of the writer, begs his wife to show love to her and treat her kindly. He refers to the Bible in which the Saviour has taught his disciples to love children.

When Joe is out at work, Mrs Thompson spends the day with Maggie and develops compassion for her. Gradually, she grows fond of her. The Thompsons end up keeping Maggie and she becomes a blessing. Mrs Thompson who is childless had become irritable and ill-tempered due to her loneliness. Now the sweetness of that sick child has changed Mrs Thompson completely. She has become a loving and caring person. Maggie is like a honey to her soul. She carries Maggie in her heart as well as in her arms. Maggie has proved to be an angel in disguise.

Thus the writer conveys the message that not only does Maggie need the care of another to survive, but Mrs Thompson also needs Maggie to lead a happy and purposeful life.

An Angel in Disguise Character Sketch

1. MAGGIE

tragically crippled young girl
unable to leave her bed, weak and helpless
symbolizes innocence
turns out to be a blessing for Mrs Thompson
transforms the irritable Mrs Thompson and makes her a happy and compassionate person
polite and humble
an angel in disguise

Maggie, the youngest child of her family, is a crippled girl. She has injured her spine after her fall from a window two years ago. She is unable to leave her bed and is dependent on the others. She is poor and helpless. She is so weak that she cannot leave her bed unless carried in someone’s arms. Maggie symbolizes innocence and helplessness because she literally cannot survive without the love and care of someone else. Maggie turns out to be a blessing in the Thompson house because she gives Mrs Thompson a purpose. She brings joy into the sad life of Thompsons.

In fact ‘an angel had come into his (Thompson’s) house, disguised as a sick, helpless child, and filled all its dreary chambers with the sunshine of love’. She talks a little but conveys her despair when she says something. After losing her family, she says : “O, Mr Thompson ! don’t leave me here all alone !” Though it is a short sentence, it has a very specific emotional colouring. She is very polite and humble. She always says ‘sir’ when she talks to Joe Thompson. In fact, Maggie’s character illustrates the main theme of the story that humans cannot survive without other humans to love and care for them.

2. MRS THOMPSON

childless
cruel, harsh, having no compassion
Maggie, the crippled child transforms her
finds purpose and direction in life
was irritable and ill-tempered before Maggie comes into her life
no longer irritable, but loving and caring woman
leads a happy and pleasant life

Mrs Thompson, who happens to be childless, is a woman without compassion, kindness and sympathy. She is not a woman of saintly temper. She is first introduced as a very cruel, harsh character with little compassion for Maggie. When her husband, Joe Thompson, enters his home with Maggie in his arms, Mrs Thompson is enraged and says why he has brought that ‘sick brat’ into their home. But after spending time with Maggie, Mrs Thompson is transformed and decides to take care of Maggie for a few more days, which eventually turn into the rest of her life.

Mrs Thompson ends up falling in love with Maggie and finds purpose in taking care and looking after her. Before Maggie comes it was dark and cold and miserable there for a long time just because Mrs Thompson had nothing to love and care for except herself, and so became sore, irritable and ill-tempered, and self-afflicting in the desolation of her woman’s nature. Now the sweetness of that sick child transforms her. She is no longer irritable and ill-tempered. She is a loving and caring person. The sweetness of Maggie is like honey to her soul, and she carries her in her heart as well as in her arms.

3. JOE THOMPSON

the wheelwright
a kind-hearted person
rough in exterior but tenderhearted from inside
loves to spend time with children
takes pity on Maggie, the crippled child
lifts her in his arms and carries her to his home
exhorts his wife to be kind and loving
his words have the desired effect on his wife

Joe Thompson, the wheelwright, is a loving, caring and kind-hearted person. He loves children and likes their company. Though rough in exterior, he has a tender heart. He is a strong man, but the experience with Maggie touches him greatly, and that is why he cries a little bit when the youngest Maggie, the crippled child, is left alone because nobody wants to deal with her disability. He takes pity on Maggie and decides to take her with him. For a while, he is puzzled because he knows that his wife would not accept her. But he soon resolves his conflict and takes her along with him.

He lifts her in his strong arms and takes her to his home. He has a great understanding of his wife. When she asks why he has brought that ‘sick brat’ to her home, he does not reply, but gives her a look that is pleading and cautionary. Then to calm his wife down, he refers to the Bible in which it is mentioned that the Saviour rebuked the disciples who did not receive and love the children. He exhorts his wife to be kind to her and treat her gently. The voice of this strong, rough man shakes and he turns his head away to hide his moist eyes. His words have the desired effect and a soft feeling creeps into his wife’s heart.

An Angel in Disguise Critical Appreciation

Third Person Narrative
The story is narrated in the third person by an omniscient narrator. The author introduces his characters and comments on them, as for example, he says about the drunken woman : “This woman had been despised, scoffed at, and angrily denounced by nearly every man, woman and child in the village.” The narrator has a pity for the children of the dead woman, especially Maggie, due to her predicament of being an orphan whom everyone is reluctant to take in. The narrator demonstrates his tender feelings for Maggie when he describes her as having ‘sad eyes’, and a patient face.

Purpose
The author wrote this story in order to illustrate the importance of caring and loving. Not only does Maggie need the care and love of another to survive, but Mrs Thompson also needs Maggie as someone to care and love for to live a happy purposeful life. This displays that humans are interdependent, and cannot live in isolation. The author proves that love is essential for human survival.

Tone
The tone of the story is a melancholy one. A woman whom none cared about previously has passed away, and the townspeople suddenly act as if they were in mourning. This tone continues as the narrator reveals that the children are orphans, and none of the townspeople is willing to take little Maggie in. Once Joe Thompson takes Maggie to his house, the tone shifts from melancholy to hope. Finally, when Mrs Thompson realizes that Maggie is bringing the couple happiness, the tone ultimately becomes optimistic.

Message
The story gives a message to alcoholics and those who discriminate against disabled persons. It shows the tragedy that alcohol can inflict on a family. It also targets those who have a bias against the disabled because it illustrates the joy and love that disabled people can bring to one’s life.

Language
The writer uses carefully planned complex sentences with powerful vocabulary in order to lay emphasis on the importance of loving and caring for others. He makes use of literary devices to make his style rich, literary and artistic. He makes use of personification in the following line: ‘Death touches the spring of our common humanity.’ The use of oxymoron ‘precious burden’ in the line ‘she carried her in her heart as well as in her arms, a precious burden’ is noteworthy.

Angel in disguise meaning

What is X Percent of Y Calculator

July 29, 2020 by worksheetsbuddy_do87uk

What is X Percent of Y Calculator helps you determine the value of R given the values of Y and Percentage X.

What is X percent of Y?

Written as an equation: R = X% * Y
The ‘what’ is R that we want to solve for
Remember to first convert percentage to decimal, dividing by 100
Solution: Solve for Y using the percentage formula
R = X% * Y

How to calculate percentage of a number. Use the percentage formula: X% * Y = R

Example 1: What is 10% of 150?

Convert the problem to an equation using the percentage formula: X% * Y = R
X is 10%, Y is 150, so the equation is 10% * 150 = R
Convert 10% to a decimal by removing the percent sign and dividing by 100: 10/100 = 0.10
Substitute 0.10 for 10% in the equation: 10% * 150 = R becomes 0.10 * 150 = R
Do the math: 0.10 * 150 = 15
R = 15
So 10% of 150 is 15
Double-check your answer with the original question: What is 10% of 150? Multiply 0.10 * 150 = 15

Take the help of What is x percent of y calculator an online math tool that calculates 10% of 150 easily along with a step by step solution detailing how the result 15 arrived.

Example 2: What is 10% of 25?

Written using the percentage formula: R = 10% * 25
First convert percentage to a decimal 10/100 = 0.1
R = 0.1 * 25 = 2.5
So 10% of 25 is 2.5

What is X Percent of Y Calculator Examples:

Percentage Tips And Tricks

Now let’s go through the short tricks that you can use to find the Percentage of any number in less time:

Q1. What is 5% of 1220?

– 10% of 1220 is 122.
– You know that 5% is half of 10%. So, half of 122 is 61.

Q2. What is 15% of 400?

– 10% of 400 is 40.
– As you know that 5% is half of 10%. So, 5% of 400 = 40/2 = 20.
– So add 10% of 400 and 5% of 400 = that will give 15% of 400 which is 40 + 20 = 60.
– Another method is that you just multiply 15 with 4. Forget about two zeros since they will be canceled by 100.

Q3. How to calculate 85% of a number?

– Number – (15% of Number) = Answer

Q5. What is 20% of 400?

– Just multiply 2 with 40 = 80. Forget about one zero present in 20 and 400 each, because two zeros will be canceled with 100.

Q4. What is 3% of 400?

– 1% of 400 is 4.
– Multiply 1% with 3 to get (3% of 400) = 4 * 3 = 12.

Know the fractional numbers as a percentage:

One-third = 1/3 of 100%	33%
One-fouth = 1/4 of 100%	25%
One-third = 1/5 of 100%	20%
One-sixth = 1/6 of 100%	17.5% (half of 1/3)
One-sixth = 1/7 of 100%	14.28%
One-eight = 1/8 of 100%	12.5% (half of 1/4)
One-ninth = 1/9 of 100%	11.11%

What is X Percent of Y Important Calculations

X % of Y	Result (R)
10 % of 1000000	100000
10 % of 5000	500
3.5 % of 150000	5250
20 % of 250	50
15 % of 200	30
15 % of 1000	150
10 % of 3000	300
3 % of 50000	1500
30 % of 3000	900
30 % of 1300	390
10 % of 500	50
30 % of 500	150
3 % of 3000	90
2 % of 5000	100
20 % of 200	40
15 % of 30000	4500
25 % of 1000	250
8 % of 100	8
80 % of 30	24
5 % of 4000	200
30 % of 1000	300
0.7 % of 100	0.7
0.3 % of 10000	30
30 % of 600	180
2 % of 10000	200
0.25 % of 10000	25
10 % of 50000	5000
10 % of 6000	600
5 % of 1500	75
5 % of 2500	125
20 % of 150	30
30 % of 400	120
10 % of 300	30
30 % of 500	150
20 % of 240	48
25 % of 180	45
3 % of 1000	30
12 % of 50	6
10 % of 100000	10000
6 % of 1000	60
8 % of 10000	800
3 % of 60000	1800
3 % of 40000	1200
15 % of 1200	180
20 % of 700	140
3 % of 4000	120
0.2 % of 10000	20
20 % of 800	160
3 % of 1500	45
20 % of 1300	260
70 % of 500	350
1.5 % of 100	1.5
20 % of 20000	4000
15 % of 240	36
0.5 % of 20000	100
20 % of 70000	14000
20 % of 100000	20000
40 % of 50	20
0.2 % of 10000	20
25 % of 400	100
10 % of 180	18
30 % of 450	135
5 % of 500	25
30 % of 7000	2100
10 % of 10	1
40 % of 55	22
0.3 % of 1800	5.4
10 % of 4000	400
5 % of 20000	1000
10 % of 1000	100
5 % of 60000	3000
10 % of 300000	30000
35 % of 1000	350
20 % of 360	72
5 % of 150000	7500
0.3 % of 50000	150
0.25 % of 100	0.25
3 % of 2500	75
30 % of 800	240
3 % of 30000	900
5 % of 10000	500
5 % of 100	5
30 % of 4000	1200
10 % of 100	10
80 % of 150	120
7 % of 5000	350
15 % of 350	52.5
30 % of 20000	6000
25 % of 160	40
0.3 % of 40000	120
12 % of 50000	6000
20 % of 3000	600
4 % of 200	8
0.25 % of 1500	3.75
10 % of 20000	2000
75 % of 50	37.5
0.2 % of 2000	4
0.3 % of 15000	45
0.25 % of 5000	12.5

Everyday Applications Of Percentage Formula

Calculation of percentage has numerous uses in our everyday lives. Some of them are listed below:

Used to express fractions in a simple way and comparison of fractions.
Percentage, in the form of discounts, is used to advertise products.
It is used to express interest charged for loans or investments.
Change in percentage like increase and decrease is used to describe profit or losses suffered by companies.
Expressed as commissions, which is a percentage of the total sales, offered to the salesperson.

We hope that you have understood What is X Percent of Y Calculator. If you have any doubts, then you can post your queries in the comment section below. We will get back to you as soon as possible. For more information, stay tuned

Playing With Numbers Worksheets for Class 6 Maths

March 30, 2020March 29, 2020 by Rajashekhar

CBSE Notes for Class 6 Maths Chapter 2 Playing With Numbers Summary is highly helpful for students who want to understand all the exercise questions. NCERT Class 6 Maths Notes and Practice Problems have been reviewed by our best Mathematics teachers. We have provided detailed notes and all the solutions for NCERT Maths class 6 Playing With Numbers. so that you can solve the questions in class 6 mathematics textbook seamlessly. CBSE worksheets for class 6 Maths are available at free of cost to all the students.

Board: Central of Secondary Education
Class: Class 6
Subject: Maths
Chapter Name: Playing With Numbers.

Factors and Multiples:
Factor: A factor of a number is an exact divisor of that number.
In other words, a factor of a number is that number which completely divides the number without leaving a remainder.
Each of the numbers 1, 2, 3, 4, 6 and 12 is a factor of 12. However, none of the numbers 5, 7, 8, 9, 10 and 11 is a factor of 12.

Multiple: A multiple of a number is a number obtained by multiplying it by a natural number.
If we multiply 3 by 1, 2, 3, 4, 5, 6, , we get
3 × 1 = 3, 3 × 2 = 6, 3 × 3 = 9, 3 × 4 = 12, 3 × 5 = 15, 3 × 6 = 18,
Thus, 3, 6, 9, 12, 15, 18, are multiples of 3.
Every multiple is equal to or greater than the given number.

Perfect Number:
Factors of 6 are 1, 2, 3 and 6
Now, the sum of the factors of 6
= 1 + 2 + 3 + 6 = 12 = 2 times of 6
Factors of 28 are 1, 2, 4, 7, 14 and 28
Now, the sum of the factors of 28 = 1 + 2 + 4 + 7 + 14 + 28 = 56 = 2 times 28
The numbers like 6 and 28 are called perfect numbers.
A number is called a perfect number if the sum of all its factors is equal to twice the number.
12 is not a perfect number.
Factors of 12 are: 1, 2, 3, 4, 6, 12
Now the sum of factors of 12 = 1 + 2 + 3 + 4 + 6 + 12 = 28 is not equal to 2 12 = 24

Even Numbers: All multiples of 2 are called even numbers.
We know that 2, 4, 6, 8, 12, 14, are multiples of 2.
Hence, 2, 4, 6, 8, 10, 12, 14, are even numbers.
Clearly, a number is even if is divisible of 2 or 2 is a factor of it.

Odd Numbers: Numbers which are not multiples of 2 are called odd numbers.
Clearly, 1, 3, 5, 7, 9, 11, 13, 15, are odd numbers.
Also, a number is either even or odd. A number cannot be both even as well as odd.
In order to list all factors of a number. We may follow the following procedure.

Prime and Composite Numbers:

Prime numbers: Numbers with only two factors, 1 and the number itself, are known as prime numbers. Examples are 2, 3, 5, 7, 11, 13,

Composite Numbers: Numbers with more than two factors are called composite numbers.
Examples are 4, 6, 8, 9, 10, 12,
Number 1 is neither prime nor composite.

	TEST OF DIVISIBILITY
No.	Divisibility Test	Examples
2	Unit digit should be 0 or even	4096, 23548 as they end with 6 and 8 i.e., even numbers
3	The sum of digits of no. should be divisible by 3.	2143251, sum of the digits is 18 and it is divisible by 3
4	The no formed by last 2 digits of given no. should be divisible by 4.	548, here 48 ÷ 4 = 12 and it is divisible by 4
5	Unit digit should be 0 or 5.	4095 and 235060 as they have 5, 0 at unit places.
6	No should be divisible by 2 & 3 both.	753618, sum of the digits is 30 and it is divisible by 2 and 3.
8	The number formed by last 3 digits of given no. should be divisible by 8.	5432, here 432 is divisible by 8
9	Sum of digits of given no, should be divisible by 9.	125847, sum of the digits is 27 and it is divisible by 9
11	The difference between sums of the digits at even & at odd places should be zero or multiple of 11.	9582540, here sum of odd places- sum of even places (22 – 11 = 11) and 11 is a divisible by 11
25	Last 2 digits of the number should be. 00, 25, 50 or 75.	2500, 2550 etc

H.C.F. & L.C.M.

H.C.F. (Highest Common Factor):
The greatest number which divides all the given numbers is called Highest Common Factor (H.C.F.). e.g., 18 and 30 are the given numbers 6 is the only greatest number which divides both 18 and 30 exactly

NOTE :-
The product of two numbers a and b is equal to the product of their L.C.M. and H.C.F.
a × b = H.C.F. × L.C.M.
Product of ‘n’ number = (H.C.F. of each pair)^{n – 1} × L.C.M. of n pair

L.C.M. (Least Common Multiple):
The least number which is exactly divisible by all the given numbers is Least Common Multiple 24 is only least common multiple of 6, 8 and 12
Ex. L.C.M. of 6, 8 and 12 is 24

Solved Examples

Problem 1.
Find the least number which when divided by 20, 25, 35 and 40 leaves remainder 14, 19, 29 and 34 respectively
Sol.
(20 – 14) = 6, (25 – 19) = 6, 35 – 29 = 6 40 – 34 = 6= r
Required number = L.C.M. of (20, 25, 35 and 40) – 6 = 1400 – 6 = 1394

Problem 2.
Find the least number which when divided by a, b and c leaves the same remainder ‘r’ in each case
Sol:
Let L.C.M. of a, b and c = M
Required number = M + r

Problem 3.
The traffic lights at three different road crossing change after every 48 sec, 72 sec and 108 sec respectively. If they all change simultaneously at 8 : 20 : 00 hours, then at what time will they again change simultaneously?
Sol.
Interval of change = (L.C.M. of 48, 72, 108) sec = 432 sec
So the light will again change simultaneously
after every 432 seconds i.e., 7min 12 sec.
Hence next simultaneous change will take place at 6 : 27 : 12 hrs.

Problem 4.
Find the greatest number that will divide 148, 246, 623 leaving remainders 4, 6 and 11 respectively
Sol.
Required No. = H.C.F. of (148 – 4), (246 – 6) and (623 – 11)
= H.C.F. of (144, 240 and 612)

H.C.F. = 12
Required No. =12

H.C.F & L.C.M of Fractions

Ex.
L.C.M. of two distinct natural numbers is 211, what is their H.C.F.?
Sol.
211 is a prime number, so there is only one pair of distinct numbers possible whose L.C.M. is 211, i.e., 1 and 211, H.C.F. of 1 and 211 is 1.

Ex.
Find number of prime factors in 2²²² × 3³³³ × 5⁵⁵⁵Sol.
No. of prime factors = 222 + 333 + 555 = 1110

Multiple Choice Questions

Problem 1.
Which of the following numbers is a perfect number?
(A) 4
(B) 12
(C) 8
(D) 6

Problem 2.
Which of the following are not twin-primes?
(A) 3, 5
(B) 5, 7
(C) 11, 13
(D) 17, 23

Problem 3.
Which of the following are co-primes?
(A) 8, 10
(B) 9, 10
(C) 6, 8
(D) 15, 18

Problem 4.
Which of the following is a prime number?
(A) 263
(B) 361
(C) 323
(D) 324

Problem 5.
The number of primes between 90 and 100 is
(A) 0
(B) 1
(C) 2
(D) 3

Problem 6.
Which of the following numbers is a perfect number?
(A) 16
(B) 8
(C) 24
(D) 28

Problem 7.
Which of the following is a prime number?
(A) 203
(B) 139
(C) 115
(D) 161

Problem 8.
The total number of even prime numbers is
(A) 0
(B) 1
(C) 2
(D) unlimited

Problem 9.
Which one of the following is a prime number?
(A) 161
(B) 221
(C) 373
(D) 437

Problem 10.
The least prime is
(A) 1
(B) 2
(C) 3
(D) 5

Problem 11.
Which one of the following number is divisible by 3?
(A) 27326
(B) 42356
(C)73545
(D) 45326

Problem 12.
Which of the following numbers is divisible by 4?
(A) 8675231
(B)9843212
(C) 1234567
(D) 543123

Problem 13.
Which of the following numbers are divisible by 6?
(A) 672
(B) 813
(C) 7312
(D) 1236
(E) 4314
(F) 689
(G) 263
(I) 8135
(J) 7236
(H) 164

Problem 14.
From the following, find the numbers divisible by 8.
(A) 328
(B) 4728
(C) 8256
(D) 9096
(E) 6324
(F) 8004
(G) 5368
(H) 6072
(I) 4568
(J) 4821

Problem 15.
From the following, find the numbers divisible by 9.
(A) 8163
(B) 7214
(C) 8353
(D) 6345
(E) 1584
(F) 3617
(G) 6273
(H) 8001
(I) 4375
(J) 8931

Problem 16.
Identify the numbers divisible by 10.
(A) 29
(B) 430
(C) 89
(D) 77
(E) 120
(F) 33
(G) 17908
(H) 3640

Problem 17.
Identify the numbers divisible by 11.
(A) 71412
(B) 376277
(C) 6116
(D) 86124
(E) 643214
(F) 20438
(G) 48295
(H) 14909
(I) 97526
(J) 563761

Problem 18.
The HCF of two consecutive odd numbers is
(A) 1
(B) 2
(C) 0
(D) non-existant

Problem 19.
The HCF of an even number and an odd number is
(A) 1
(B) 2
(C) 0
(D) non-existant

Problem 20.
The LCM of 24, 36 and 40 is
(A) 4
(B) 90
(C) 360
(D) 720

We hope the NCERT Solutions for Class 6 Maths Chapter 2 Playing With Numbers help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 2 Playing With Numbers, drop a comment below and we will get back to you at the earliest.

Knowing Our Numbers Worksheets for Class 6 Maths

March 30, 2020May 9, 2019 by Rajashekhar

CBSE Notes for Class 6 Maths Chapter 1 Knowing our Numbers Summary is highly helpful for students who want to understand all the exercise questions. NCERT Class 6 Maths Notes and Practice Problems have been reviewed by our best Mathematics teachers. We have provided detailed notes and all the solutions for NCERT Maths class 6 Knowing our Numbers. so that you can solve the questions in class 6 mathematics textbook seamlessly. CBSE worksheets for class 6 Maths are available at free of cost to all the students.

Board: Central of Secondary Education
Class: Class 6
Subject: Maths
Chapter Name: Knowing Our Numbers

Comparing Numbers :
As we have done quite a lot of this earlier, let us see if we

How many numbers can we make?
Suppose, we have four digits 1, 2, 3, 4. Using these digits we want to make different 4-digit numbers such that no digits are repeated in a number. Thus, 3241 is allowed but 1123 is not. The greatest number is 4321 and the smallest is 1234.
Ex: Use the given digits without repetition and make the greatest and smallest 4-digit numbers.
(a) 2, 8, 6, 1
(b) 3, 0, 7, 4
(c) 8, 9, 2, 3
(d) 5, 0, 8, 2
Sol:
(a) Greatest number is 8621, Smallest number is 1268
(b) Greatest number is 7430, Smallest number is 3047
(c) Greatest number is 9832, Smallest number is 2389
(d) Greatest number is 8520, Smallest number is 2058

Ascending order:
Ascending order means arrangement from the smallest to the greatest.
Ex. 847, 9754, 8320, 571
Sol. 571, 847, 8320, 9754

Descending order:
Descending order means arrangement from the greatest to the smallest.
Ex. 847, 9754, 8320, 571
Sol. 9754, 8320, 847, 571

Introducing 10,000:
We observe that :
Greatest single digit number+1 = smallest 2-digit number
9 + 1 = 10
Greatest 2-digit number + 1 = smallest 3-digit number
99 + 1 = 100
Greatest 3-digit number + 1 = smallest 4-digit number
999 + 1 = 1000
Greatest 4-digit number + 1 = smallest 5-digit number
9999 + 1 = 10,000

Remember :
1 hundred = 10 tens
1 thousand = 10 hundreds = 100 tens
1 lakh = 100 thousands = 1000 hundreds
1 crore = 100 lakhs = 10,000 thousands

Use of Commas:
1. Indian System of Numeration: In our Indian System of Numeration we use one, tens, hundreds, thousands and then lakhs and crores. The first comma comes after hundreds place (three digits from the right) and marks thousands. The second comma comes two digits later (five digits from the right). It comes after ten thousands place and marks lakh. The third comma comes after another two digits (seven digits from the right). It comes after ten lakh place and marks crore.
For example, 5, 78, 21, 623

2. International System of Numeration: In the International System of Numeration, as it is being used we have ones, tens, hundreds, thousands and then millions. One million is a thousand thousands. Commas are used to mark thousands and million. It comes after every three digits from the right. The first comma mark thousands and the
next comma marks millions.
For example, 57, 821, 623

Remember:
10 millimetres = 1 centimetre
1 metre = 100 centimetres = 1000 millimetres
1 kilometre = 1000 metres = 10,00,000 millimetres
1 gram = 1000 milligram
1 litre = 1000 millilitres.

Solved Examples:
Ex 1.
Insert commas suitably and write the names according to Indian System of Numeration :
(a) 87595762
(b) 8546283
(c) 99900046
(d) 98432701
Sol:
(a) 8,75,95,762 = Eight crore seventy-five lakh ninety-five thousand seven hundred sixty two.
(b) 85,46,283 = Eighty-five lakh forty-six thousand two hundred eighty-three.
(c) 9,99,00,046 = Nine croe ninety-nine lakh forty six.
(d) 9,84,32,701 = Nine crore eighty-four lakh, thirty-two thousand seven hundred one.

Ex 2.
Insert commas suitably and write the names according to International System of Numeration :
(a) 78921092
(b) 7452283
(c) 99985102
(d) 48049831
Sol.
(a) 78,921,092 = Seventy-eight million, nine hundred twenty-one thousand, ninety-two.
(b) 7,452,283 = Seven million four hundred fifty-two thousand two hundred eighty-three.
(c) 99,985,102 = Ninety-nine million nine hundred eighty-five thousand, one hundred two.
(d) 48,049,831 = Forty-eight million forty-nine thousand eight hundred thirty one.

Ex 3.
Population of Kota was 5,48,985 in the year 1995. In the year 1996 it was found to be increased by 1221. What was the population of the city in 1996?
Sol.
Population of the city in 1996
= Population of the city in 1995 + Increase in population
= 5,48,985 + 1221

Types of Numbers:

(i) Natural Numbers: (N)
Set of all counting numbers from 1 to+∞,N = {1,2,3,4,… ∞}.
(ii) Whole Numbers: (W)
Set of all natural numbers including zero, W = {0,1,2,3,4,… }
(iii) Integers:
Set of all positive and negative of natural numbers including zero from – ∞, to + ∞, I or Z = {…,-3, -2, -1, 0, 1, 2, 3,
…}.
Positive integers {1, 2, 3, 4 }
Negative integers { -3, -2, -1}
(iv) Rational Numbers:
These are real numbers which can be expressed in the form of p/q, where p and q are integers and q ≠ 0. e.g., 2/3, 37/15, /17/19 etc.
All the integers are rational numbers, because every integer can be expressed in the form p/q. For example, the integers

Note:
1. All the fractions are rational numbers.
2. Every natural number is a rational number.
3. Every whole number is a rational number.
4. Every integer is a rational number.
5. Every fraction is a rational number.

Density property of rational numbers:
Between any two different rational numbers, there are infinitely many rational numbers. To find many rational numbers between two given distinct rational numbers, use the following method.

Here q₁, q₂, q₃, q₄……….. are the rational numbers between two distinct rational numbers a and b.

Ex 1.
Find three rational numbers between 1/5 and 1/3.
Sol.
Let q₁, q₂, q₃ be the three required rational numbers. Then

Comparison of two rational numbers:
Using the arithmetical process:

Express each rational number with a positive denominator
Find LCM of the positive denominators
Express each of the given rational numbers with LCM as the common denominator
The number having greater numerator is greater

To compare two rational numbers p/q and r/s. we compare the products s × p and q × r and define their inequality as under

Properties of rational number:
Decimal representation of a rational number:
We can represent a rational number as a decimal number by the long division process. We have three types of decimals. They are

Terminating decimals
Non-terminating and repeating decimals
Non-terminating and non-repeating decimals
Defination of terminating, non-terminating, recurring & non-recurring.

Ex.2:
√2, √3 etc.
Converting a decimal number into an equivalent rational number in the form of a/b
We can divide this into two parts :
Case – 1: When the decimal number is a terminating number. Express 0.3 as a rational number.
Sol.
0.3 = 3/10

Introduction of irrational numbers:
Every rational number can be expressed either in terminating decimal form or non-terminating repeating (or periodic) decimal form. Consider the decimal number 0.1010010010001 We can see that no group of digits is repeating in a cyclic manner. So we have numbers whose decimal representation is neither terminating nor repeating. There are a number of such decimals. Such numbers are called irrational numbers.

Note:
A number is an irrational number, if it has a non-terminating and non-cyclic repeating decimal representation.

Real Numbers:
The set of rational numbers together with the set of irrational numbers are called ‘Real Numbers’, denoted by “R”

Whole Numbers:
The natural numbers along with zero form the collection of whole numbers. 0, 1, 2, 3, 4, 5, are whole numbers.

Successor:
Success of every number comes just after the number. Therefore, the successor of every number is obtained by adding 1 to the number.
For example: successor of 98 = 98 + 1 = 99

Predecessor:
Predecessor of every number comes just before the number.
For example: predecessor of 98 = 98 – 1 = 97

Representation of Numbers on Number Line:
Every number on number line represents position from its greater and smaller numbers. Negative, positive, whole, natural, prime, etc. All are represented on number line.
Numbers on number line are represented by the following:
Step 1:
Draw a line and mark a point zero on it.
Step 2:
Distance between the numbers is always equal. Therefore, the length of number line should be divided into required into required number of interval.
Step 3:
The arrow mark at the far end point of number line, indicates infinitive.

Ex:
Representation of whole numbers from 0 to 10 on number line.
Step 1:
Draw a horizontal line and mark a point 0 on it.
Step 2:
Mark another point and divide the distance among them into 10 equal parts.
Step 3:
Write numbers 1 to 10 at each division.

Properties of whole numbers:
1. Addition:
Sum of any two whole numbers is always a whole number i.e. the collection of whole numbers is closed under addition.
For example : 2 + 3 = 5

2. Substraction:
Substraction of any two whole number is not always a whole number.
For example : 3 – 2 = 1 (whole number). But 2 – 3 = -1 (not whole number)

3. Multiplication:
Multiplication of any two whole number is always a whole number.
For example : 2 × 3 = 6, 2 × 0 = 0.

4. Division:
Division of any two whole number is not always a whole number.
For example : 6/3 = 2 (is whole number) 2/3 = (not a whole number)
3/0 (not possible)

Solved Examples:

Problem 1.
Add the following:
347 + 578 + 153
Sol.
We can group the numbers in different ways.
As 347 + 153 = 500,
So, we can group
347 + 578 + 153 = (347 + 153) + 578 = 500 + 578 = 1078

Problem 2.
Show that:
(63 + 49) + 37 = 63 + (49 + 37)
Sol.
(63 + 49) + 37 = 112 + 37 = 149
63 + (49 + 37) = 63 + 86 = 149
(63 + 49) + 37 = 63 + (49 + 37)

Problem 3.
Solve by suitable rearrangement.
32 × 25
Sol.
32 is equal to 8 × 4
So, 32 × 25 = 8 × 4 × 25
= 8 × (4 × 25)
= 8 × 100 = 800

Problem 4.
Simplify using properties .
4 × 16 × 125
Sol.
4 × 125 = 500
So, 4 × 16 × 125 = 16 × 4 × 125 = 16 × (4 × 125)
= 16 × 500 = 8000

Problem 5.
A florist arranges 6 gladioli and 7 roses in a bouquet. Raj buys 5 such bouquets for the school annual function. What is the total number of flowers in these 5 bouquets?
Sol.
Gladioli in 5 bouquets = 5 × 6 flowers = 30 flowers Rose in 5 bouquets = 5 × 7 flowers = 35 flowers Total number of flowers in 5 bouquets = (5 × 6) + (5 × 7) = 65 flowers Another ways of solving the problem is as follows.
Flowers in one bouquets (gladioli = roses) = (6 + 7) flowers
Total number of flowers in 5 bouquets = 5 (6 + 7) flowers = 5 × 13 flowers = 65 flowers
So, 5(6 + 7) = (5 × 6) + (5 × 7)
5 × 13 = 30 + 35
65 = 65
Hence, we can conclude that if a, b and c are whole numbers,
then a × (b + c) = a × b + a × c
This property is called the distributive property of multiplication over addition.

Problem 6.
7 × (8 + 3) = 7 × 8 + 7 × 3
Sol.
We can see that multiplication is distributed between 8 and 3 when the sign is one of addition.
Let us look at another example.

6 rows of 5 squres + 6 rows of 4 squares
= 5 x 6 + 4 × 6 = 30 + 24 = 54 squares.
This is also equal to 6 rows of 9 squares.
6 × 9 = 54
So, 5 × 6 + 4 × 6 = 6 (5 + 4)
30 + 24 = 6 × 9
54 = 54

Problem 7.
There are 7 plates. Six biscuits are placed on each plate. If 4 biscuits are taken away from each plate, how many biscuits are left on the plates ? Write the mathematical statement.
Sol.
Biscuits on 7 plates = 7 × 6 = 42 biscuits
Biscuits taken away from 7 plates = 7 × 4 = 28 biscuits
Biscuits remaining = 7 × 6 – 7 × 4
= 42 – 28 = 14 biscuits
or, Biscuits left on one plate = (6 – 4) biscuits
Biscuits left on 7 plates = 7(6 – 4) biscuits
= 7 × 2 biscuits = 14 biscuits
So, the mathematical statement
7 × (6 – 4) = 7 × 6 – 7 × 4

Problem 8.
Write the place values of the bold digits in each of the following :
(a) 370345
(b) 2479034
(c) 42371509
Sol.
(a) The place value of 7 in 370345 is 70,000.
(b) The place value of 2 in 2479034 is 20,00,000.
(c) The place value of 1 in 42371509 is 1,000.

Problem 9.
Write the following numbers in expanded notation.
(a) 4,89,342
(b) 31,85,204
(c) 3,24,05,620
Sol.
(a) 4,89,342 = 4,00,000 + 80,000 + 9,000 + 300 + 40 + 2
(b) 31,85,204 = 30,00,000 + 1,00,000 + 80,000 + 5,000 + 200 + 4
(c) 3,24,05,620 = 3,00,00,000 + 20,00,000 + 4,00,000 + 5,000 + 600 + 20

Problem 10.
Write the following numbers in standard numerals.
(a) 1,00,000 + 70,000 + 8,000 + 900 + 20 + 3
(b) 70,00,000 + 2,00,000 + 4,000 + 50 + 8
(c) 5,00,00,000 + 6,00,000 + 40,000 + 3,000 + 800 + 5
Sol.
(a) 1,00,000 + 70,000 + 8,000 + 900 + 20 + 3 = 1,78,923
(b) 70,00,000 + 2,00,000 + 4,000 + 50 + 8 = 72,04,058
(c) 5,00,00,000 + 6,00,000 + 40,000 + 3,000 + 800 + 5 = 5,06,43,805

Problem 11.
Write the following numbers in words.
(a) 1,78,040
(b) 23,06,789
(c) 5,03,56,033
Sol.
(a) 1,78,040 = One lakh seventy-eigth thousand forty
(b) 23,06,789 = Twenty-three lakh six thousand seven hundred eighty-nine
(c) 5,03,56,033 = Five crore three lakh fifty-six thousand thirty-three

Problem 12.
Write the following numbers in figures.
(a) Six lakh twenty thousand eighty-seven
(b) Twenty-nine lakh forty thousand thirty-eight
(c) Three crore five lakh thirty-six thousand seven
Sol.
(a) Six lakh twenty thousand eighty-seven = 6,20,087
(b) Twenty-nine lakh forty thousand thirty-eight = 29,40,038
(c) Three crore five lakh thirty-six thousand seven = 3,05,36,007

Multiple Choice Questions

Problem 1.
If N is the set of all natural numbers and W is the set of all whole numbers then overlapped part of N represent of which one of the following number ?

(A) 1
(B) 0
(C) 2
(D) all of these

Problem 2.
From the following sets of numbers, which one of the following is the set of co primes ?
(A) {8, 16}
(B) {10, 100}
(C) {3, 5}
(D) All of these

Problem 3.

(A) irrational number
(B) Rational number
(C) Solution is zero
(D) All of these

Problem 4.
How many prime numbers are there between 100 and 125?
(A) 5
(B) 6
(C) 3
(D) All of these

Problem 5.
Two consecutive prime numbers are given, if one of them is 5 then the second number is:
(A) 3
(B) 7
(C) 9
(D) All of these

Problem 6.
If the sum of x + (y + z) = m + n then the sum of (y + z) + x is?
(A) m – n
(B) n – m
(C) m + n
(D) All of these

Problem 7.
The sum of difference of two whole numbers is a whole number then the product of two whole numbers is?
(A) Prime number
(B) Whole number
(C) Composite number
(D) All of these

Problem 8.
Simplify the (34 + 20) × 2 an find the multiplicative inverse of the resulting simplification:
(A) 2/108
(B) 1/108
(C) 3/108
(D) All of the above

Problem 9.
The division of y by x is z then the division of x by y is?
(A) z
(B) Other than z
(C) Less than z
(D) All of these

Problem 10.
How many different three digit numbers can be obtained by using the digits, 0, 1, 3 without repeating any digit in the number?
(A) 4
(B) 5
(C) 3
(D) 2

Problem 11.
Which one of the following is the smallest seven digit number having four different digits?
(A) 1230000
(B)0000123
(C) 1000023
(D) 1000032

Problem 12.
Find the value of 6 + 2[3 + 5{ 28 – 12(12 – 10)}]
(A) 56
(B) 52
(C) 53
(D) Both (A) and (C)

Problem 13.
A man gets pass for 1 year for Rs. 1500. If the man has been paid 11 payments then find the amount paid by him:
(A) Rs. 16500
(C) 17000
(C) Rs. 17500
(D) Both (A) and (C)

Problem 14.
The successor of the lowest composite number is?
(A) 6
(B) 5
(C) 3
(D) 4

Problem 15.
Smallest four digit number is divided by smallest prime number. Find the predecessor of the quotient:
(A) 500
(B) 501
(C) 499
(D) 599

Problem 16.
Which one of the following is not correct?
(A) Successor of a number can be obtained by adding 1
(B) The difference between successor and predecessor of a number is smallest composite number
(C) Successor of greatest 5 digit number is smallest six digit number and predecessor of smallest 6 digit number is greatest 5 digit number
(D) The difference between lowest natural number and whole number is 1

Problem 17.
M and N are co-prime numbers, M represents lowest odd prime number. Which one of the following is the factor of their product?
(A) 12
(B) 24
(C) 10
(D) 20

Problem 18.
8m – 145 = Greatest 5 digit number, find the value of m.
(A) 12618
(B)12718
(C) 12818
(D)12518

Problem 19.
Which on of the following is the set of the single digit prime number?
(A) {0,1,2,3,5,7}
(B) {2,3,5,7}
(C) {2,3,4,5,7}
(D) {0,1,2,51}

Problem 20.
Smallest 3 digit number is multiplied by smallest prime number and smallest composite number is added to it. Find the number
(A) 200
(B) 204
(C) 206
(D) 202

Problem 21.
A man takes rest for 10 minutes after every 30 minute, if he runs 2 km in 10 minutes, find the distance covered by him in 80 minutes:
(A) 11 km
(B) 7 km
(C) 10 km
(D) 12 km

Problem 22.
Two cars, one red coloured and another blue coloured are running in a racing competition. The track is 500 km long. Speed of red car in the beginning is 350 km/hour which reduces to 275 km/hour after running 45 minutes and speed of blue car in the beginning is 325 km/hour which increases to 350 km/hour after running 45 minutes. Which car would win the race?
(A) Red car
(B) Blue car
(C) Both will complete the race at equal time
(D) Data is insufficient to answer the question

Problem 23.
A fan rotates on its shaft around 5 times in one second. How many times does the fan rotate in a day if there was no electricity for 2 hours?
(A) 396000 times
(B) 39600 times
(C) 395000 times
(D) 398000 times

We hope the NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers, drop a comment below and we will get back to you at the earliest.